2
$\begingroup$

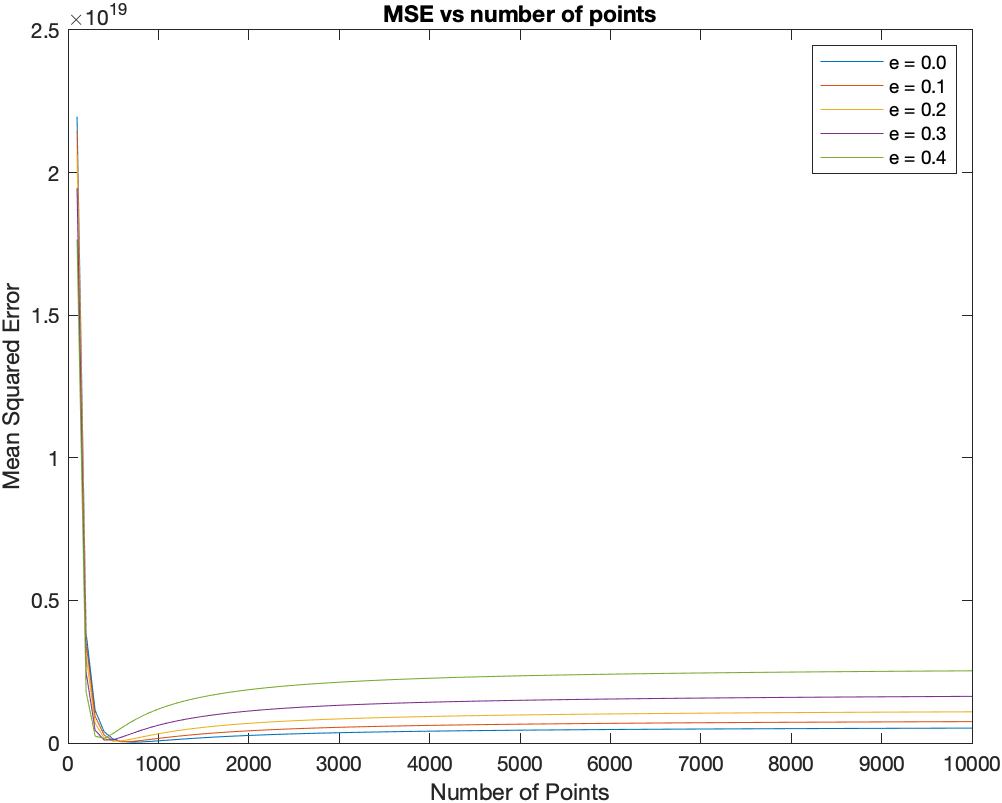
I am currently working on a project where I have used an RK4 integrator to attempt to solve the three-body problem. An interesting result that I found, was that decreasing the size of the time steps only improved accuracy until a certain point where the accuracy started to decrease. Is this normal for RK4 integrators or have I done something wrong? if this is normal, can anyone explain why this behaviour occurs?

plot of mean squared error vs number of points used

Improve this question
$\endgroup$
5
  • 6
    $\begingroup$ While not for RK, this answer of mine should be relevant. $\endgroup$
    – Anton Menshov ♦
    Commented May 30, 2022 at 6:46
  • 3
    $\begingroup$ How did you get your solution to the three body problem? Are you sure it's "correct"? It's a chaotic system so after any reasonable amount of time, all solutions will have O(1) error. $\endgroup$
    – Chris Rackauckas
    Commented May 30, 2022 at 15:28
  • 1
    $\begingroup$ @AntonMenshov 's comment should be the accepted answer. $\endgroup$
    – MPIchael
    Commented May 31, 2022 at 12:05
  • 1
    $\begingroup$ Another contribution to the topic: math.stackexchange.com/questions/3235194/…. You can reduce the increase of the error to the right by employing Kahan or compensated summation of the step updates. This, roughly, will give an error curve similar to $\max(h^p, \mu, \mu^2/h)$, realistically each term will have a problem-specific constant. $p$ is the order of the method, $\mu$ the machine constant of floating point precision. $\endgroup$
    – Lutz Lehmann
    Commented May 31, 2022 at 18:10
  • $\begingroup$ Do you have a code snippet to replicate your simulations ? $\endgroup$
    – Laurent90
    Commented Jun 3, 2022 at 16:25

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.