Derivative of the inverse of the Right Cauchy-Green Deformation Tensor wrt itself

Question

In continuum mechanics, we define the Right-Cauchy-Green Deformation Tensor as

$\boldsymbol{C}=\boldsymbol{F}^T\boldsymbol{F}$

I want to compute $\frac{\partial \boldsymbol{C}^{-1}}{\partial \boldsymbol{C}}$.

In the Wikipedia Article covering Tensor Derivatives, it says that in index form $ \left(\frac{\partial \boldsymbol{C}^{-1}}{\partial \boldsymbol{C}}\right)_{IJKL} = -C^{-1}_{IK}C^{-1}_{LJ}$. (Correct?)

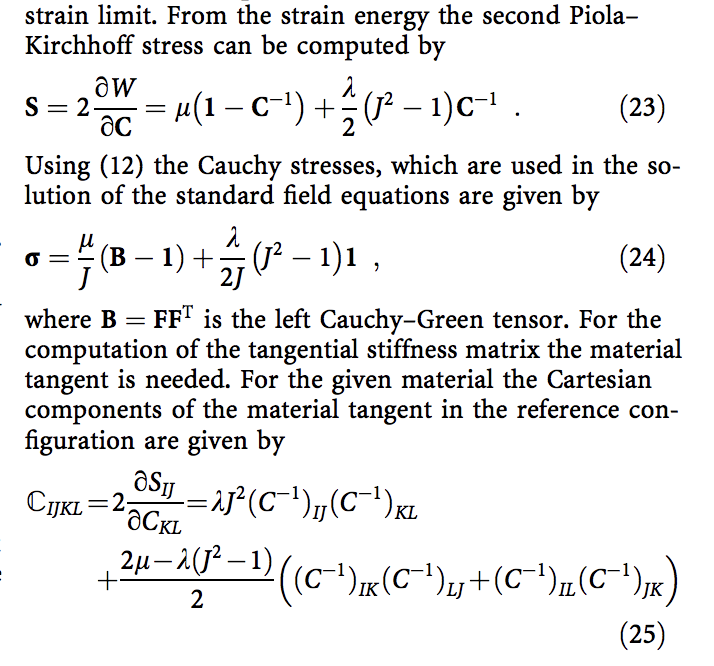
What I'm confused is this part here found in a paper regarding continuum mechanics (but also appears in many other places) :

Note that I am trying to figure out the step where we go from the Second-Piola-Kirchhoff to the Material Tangent Tensor. The Second Piola-Kirchhoff Stress $\boldsymbol{S}$ is related to the Material Tangent Tensor $\mathbb{C}$ by

$\mathbb{C} = 2\frac{\partial \boldsymbol{S}}{\partial \boldsymbol{C}}$.

and we should be able to use the tensor derivative given in the wikipedia article to get what we need.

What I don't get is how the derivative $\left(\frac{\partial \boldsymbol{C}^{-1}}{\partial \boldsymbol{C}}\right)_{IJKL}$ got splitted into two parts, $(IKLJ)$ and $(ILJK)$. There is only one term in the Wikipedia article.

Can somebody correct my misunderstanding?

Answer 1

Nick Alger gives a nice explanation. Here is another one, possibly slightly simpler because it avoids the "should stay roughly the same" part.

Let's say you want to compute the derivative of any matrix function $X=X(C)$ with regard to entry $C_{ij}$: $$ \frac{\partial X}{\partial C_{ij}}. $$ In other words, you ask how the matrix $X$ changes as you change $C_{ij}$ a bit. This can easily be answered by considering that $XX^{-1}=I$, independent of any of the elements of $C$. In other words, $$ \frac{\partial XX^{-1}}{\partial C_{ij}}=0 $$ because $XX^{-1}=I$ does not change if you modify $C_{ij}$. But by the product rule, you have $$ \frac{\partial XX^{-1}}{\partial C_{ij}}= X\frac{\partial X^{-1}}{\partial C_{ij}} + \frac{\partial X}{\partial C_{ij}}X^{-1} = 0 $$ and consequently $$ X\frac{\partial X^{-1}}{\partial C_{ij}} = - \frac{\partial X}{\partial C_{ij}}X^{-1} $$ or equivalently, assuming that the matrix $X$ is invertible: $$ \frac{\partial X^{-1}}{\partial C_{ij}} = - X^{-1} \frac{\partial X}{\partial C_{ij}}X^{-1}. $$ This is true for any function $X=X(C)$. In particular, it is true if you consider $X(C)=C$, in which case you get $$ \frac{\partial C^{-1}}{\partial C_{ij}} = - C^{-1} \frac{\partial C}{\partial C_{ij}}C^{-1}. $$ In component notation, this can be written as $$ \frac{\partial (C^{-1})_{kl}}{\partial C_{ij}} = - C^{-1}_{km} \frac{\partial C_{mn}}{\partial C_{ij}}C^{-1}_{nl}. $$ Now, the derivative of $C_{mn}$ with regard to $C_{ij}$ is only nonzero if $m=i,n=j$, and in that case it is in fact one. So $$ \frac{\partial C_{mn}}{\partial C_{ij}} = \delta_{mi}\delta_{nj}, $$ with the Kronecker delta symbol, which then finally yields this: $$ \frac{\partial (C^{-1})_{kl}}{\partial C_{ij}} = - C^{-1}_{ki} C^{-1}_{jl}. $$ This also explains what exactly the various indices in your formula correspond to.

Answer 2

We know that $$C C^{-1} = I$$ If we perturb C a little bit, by a matrix $X$, and recompute the inverse, the result should stay roughly the same. In symbols: $$(C + X) \left(C^{-1} + \underbrace{\frac{\partial C^{-1}}{\partial C}\cdot X}_{\text{matrix}}\right) \approx I,$$ where "$\frac{\partial C^{-1}}{\partial C}\cdot X$" is the first order correction to $C^{-1}$ due to the perturbation by $X$ (we want to determine this).

Multiply things out: $$I + C \frac{\partial C^{-1}}{\partial C}\cdot X + X C^{-1} + \underbrace{X \frac{\partial C^{-1}}{\partial C}\cdot X}_{O(X^2) \approx 0} = I.$$ Then drop the term that is quadratic in $X$ (since tends to zero much faster than terms that are linear in $X$), and solve the resulting equation for $\frac{\partial C^{-1}}{\partial C}\cdot X$. You get the following formula: $$\frac{\partial C^{-1}}{\partial C}\cdot X = -C^{-1} X C^{-1}.$$ This completely describes how to compute the directional derivative of of the map $C \mapsto C^{-1}$ in direction $X$.

Viewing $X$ as a free variable, this formula describes a 4'th order multilinear map. When written out in components, it becomes: \begin{align} \overbrace{\frac{\partial C^{-1}}{\partial C}}^{4'\text{th order tensor}} : (u,X ,v) \mapsto & -u^T C^{-1} X C^{-1} v \\ =& -\sum_{IJKL} \left( C^{-1} \right)_{IK} \left( C^{-1} \right)_{LJ} u_I X_{KL} v_J, \end{align} and hence the tensor $-\left( C^{-1} \right)_{IK} \left( C^{-1} \right)_{LJ}$ represents $\frac{\partial C^{-1}}{\partial C}$ when all quantities are expressed in a given basis.

You can make everything in this post rigorous by keeping track of the error committed at each step, then using Taylor's theorem and making a limiting argument.