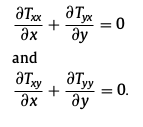I have the equilibrium equation in elasticity for a static case.i.e Div T=0. For certain implementation, I have to get the x and y component equations and then derive the weak form separately. How is it done in that case? I am aware of getting the weak form using the vector equation as such, but what I need are two separate weak form for the following equations.
-
2$\begingroup$ Umh, there is only one weak form of the system of equations. There are not two weak forms just because your system of equations consists of two equations. (This makes me wonder why you actually think that you need that. Maybe expand your question by what you want to do?) $\endgroup$– Wolfgang BangerthCommented Feb 15, 2018 at 7:06
-
$\begingroup$ Of course you can integrate each equation and apply Green's theorem. Integrate them noticing that $nx=dy/ds$ and $n_y=-dx/ds$ where $ds$ is a differential of curve. $\endgroup$– HBRCommented Feb 15, 2018 at 11:13
-
$\begingroup$ Hi Wolfgang Bangerth,I am solving a system in elasticity where the stress strain relations are implicit and hence my solver (Fenics) which usually requires formulations in weak form is unable to proceed by the conventional way. Hence, I am trying to solve the above given vector equation along with my stress strain relations by breaking down to a set of scalar differential equations. $\endgroup$– Aswin RajeevanCommented Feb 15, 2018 at 16:13
1 Answer
For your first equation you obtain the weak form integrating by parts: $$\int_\Omega\left[ \frac{\partial T_{11}}{\partial x}+\frac{\partial T_{21}}{\partial y}\right]\phi(x,y)\,dxdy=0$$
as follows
$$\int_\Omega\left[ \frac{\partial\phi}{\partial x}T_{11}+\frac{\partial\phi}{\partial y}T_{21}\right]\,dxdy=\int_\Omega\left[ \frac{\partial T_{11}\phi}{\partial x}+\frac{\partial T_{21}\phi}{\partial y}\right]\,dxdy$$
According to Green's theorem, the right hand side of the previous equation equals to: $$\int_{\partial\Omega} T_{11}\phi\,dy-\int_{\partial\Omega}T_{21}\phi\,dx$$ Since $n_x=\frac{dy}{dl}$ and $n_y=-\frac{dx}{dl}$ you can write:
$$\int_\Omega\left[ \frac{\partial\phi}{\partial x}T_{11}+\frac{\partial\phi}{\partial y}T_{21}\right]\,dxdy=\int_{\partial\Omega} \left[T_{11}\phi n_x+T_{21}\phi n_y\right]\,dl$$
You can follow the same procedure with the other equation.
-
$\begingroup$ Will such a separate formulation be identical to the normal approach with only one weak form? $\endgroup$– Pu ZhangCommented Feb 15, 2018 at 17:06
-
$\begingroup$ Actually @PuZhang you can verify that the weak formulation is the same, if you take the normal approach. $\endgroup$– HBRCommented Feb 15, 2018 at 20:56