Trying to study the error of FDM for a second order derivative versus step size.
I I calculated the coefficients and validated them, but the output has errors for small step sizes.
The
The function in question is: $ f(x) = e^{sin(x)} $
With
$$ f(x) = e^{\sin(x)} $$
With a derivative of $ f''(x) = \left(cos^2(x)-sin(x)\right)e^{sin(x)} $
The$$ f''(x) = \left(\cos^2(x) - \sin(x)\right)e^{\sin(x)} $$
The calculated FDM: $$ \frac{\partial^{(2)}f}{\partial x^{(2)}}\approx -\frac{5}{2h^2}f(x)-\frac{1}{12h^2}f(x-2h)+\frac{4}{3h^2}f(x-h)+\frac{4}{3h^2}f(x+h)-\frac{1}{12h^2}f(x+2h) $$
$$\frac{\partial^{(2)}f}{\partial x^{(2)}}\approx -\frac{5}{2h^2}f(x) - \frac{1}{12h^2}f(x-2h) + \frac{4}{3h^2}f(x-h) + \frac{4}{3h^2}f(x+h) - \frac{1}{12h^2}f(x+2h)$$
And my octaveOctave implementation:
stps = 1e-7:1e-6:3e-5;
outs = [];
x = pi/2;
for h = stps
coefficients = [-5/2 -1/12 4/3 4/3 -1/12]./h^2;
steps = [0 -2 -1 1 2].*h;
outs(end+1) = sum(coefficients .* exp(sin(x + steps)));
end
plot(stps,outs, "linewidth",1.5 , stps, ones(size(stps)).* (cos(x)^2 - sin(x))*exp(sin(x)) , "linewidth",1.5)
Which produces the following plot:
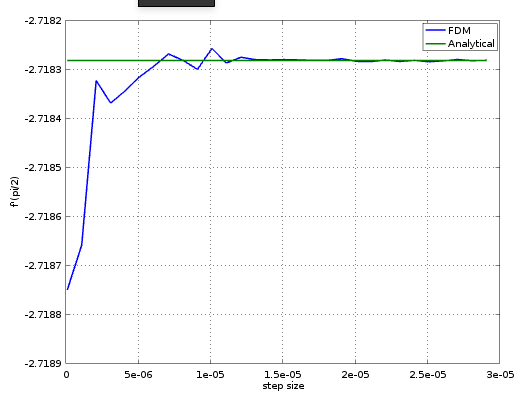
Are those rounding errors caused by the nature of floating point numbers and operations or something else?