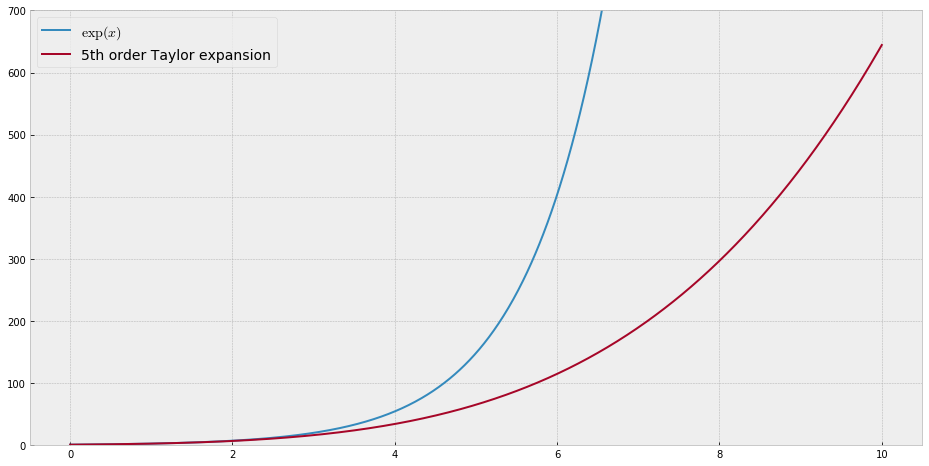
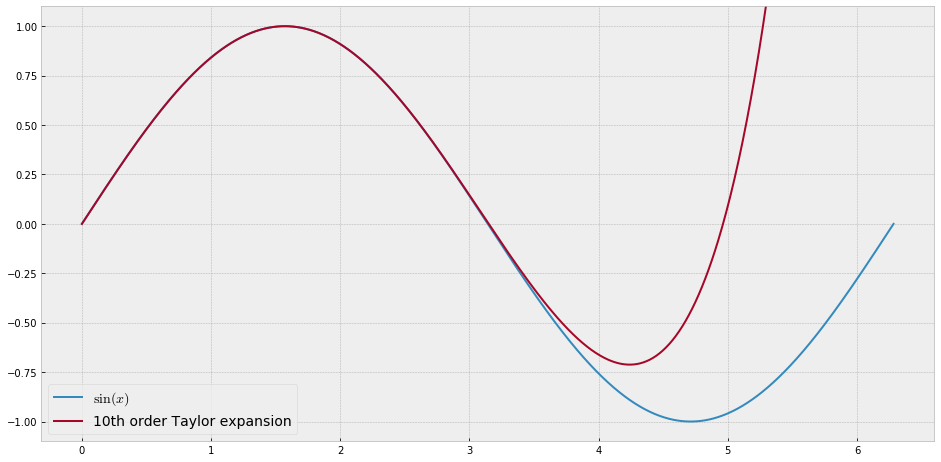
Approximation is not only made hard by the function to be approximated but by the interval in which the approximation should be a "good fit". And you should define the measure for a "good fit", i.e. what is the maximum (absolute or relative) error you wish to tolerate?
For example, you will need a huge number of terms in the Taylor series of $exp(x)$$\exp(x)$ to have a reasonable fit on the interval $[0,10]$. The same holds for periodic functions. Take $sin(x)$$\sin(x)$, for example, on the interval $[0,2\pi]$. See pictures below...