General question

I work on the plane where I have a two-dimensional shape $V$ that is cut in a collection of parts $\{V_i\}$ that do not overlap

$ V_i ~~\text{s.t.}~~ \bigcup_i \overline{V}_i = \overline{V} ~~\text{and}~~ \bigcap_i V_i = \{\emptyset\} $

I know the value of the integral of a scalar field $q(\pmb{x})$ over each part $V_i$

$ \int_{V_i} q(\pmb{x}) \, \pmb{dx} ~~ \text{known for all \(i\)} $

and I would like to get an estimation of the field $q(\pmb{x})$ for any point $\pmb{x}$ of space.

What methods can do that?

My particular case

Here is an example of the shape $V$ cut into non-overlapping (triangular) subshapes $\{V_i\}$:

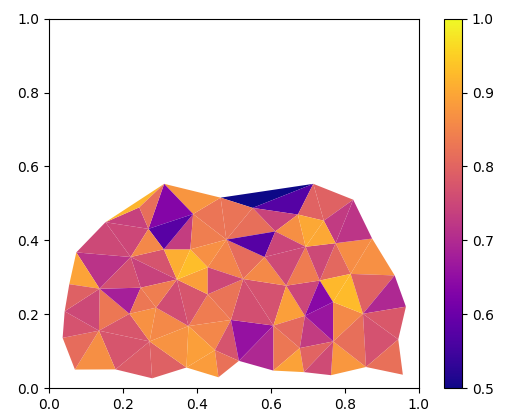
where the colors in the plot corresponds to the value of a packing factor $P(V_i)$ calculated from a characteristic function $\chi$ as $ P(V_i) := \frac{\int_{V_i} \chi(\pmb{x})}{\int_{V_i} 1} $

I would like to find a function $p$ such that $ P(V_i) = \int_{V_i} p(\pmb{x}) ~~ \forall i $
Of course, being in a general 2D case, there is no such thing as a primitive function… How can I estimate function $p$?