I'm trying to take data in numpy arrays, interpolate them and retrieve this function to differentiate/integrate at different stages.
Here is how I got here, data included:
import numpy as np
x = np.array([ 50., 55., 60., 65., 70., 75., 80., 85., 90.,
95., 100., 105., 110., 115., 120., 125., 130., 135.,
140., 145., 150., 155., 160., 165., 170., 173., 174.,
175., 176., 177., 178., 179., 180., 181., 182., 183.,
184., 185., 186., 187., 188., 189., 190., 191., 192.,
193., 194., 195., 196., 197., 198., 199., 200., 201.,
202., 203., 204., 205., 206., 207., 208., 209., 210.,
211., 212., 213., 214., 215., 216., 217., 218., 219.,
220., 221., 222., 223., 224., 225., 226., 227., 228.,
229., 230., 231., 232., 233., 234., 235., 236., 237.,
238., 239., 240., 245., 250., 255., 260., 265., 270.,
275., 280., 285., 290., 295., 300., 305., 310., 315.,
320., 325.])
y = np.array([ 1.00000000e-02, 1.00000000e-02, 1.00000000e-02,
1.00000000e-02, 1.00000000e-02, 1.00000000e-02,
1.00000000e-02, 1.50000000e-02, 2.00000000e-02,
2.50000000e-02, 3.50000000e-02, 4.00000000e-02,
5.00000000e-02, 6.00000000e-02, 8.00000000e-02,
1.05000000e-01, 1.30000000e-01, 1.70000000e-01,
2.20000000e-01, 2.75000000e-01, 3.50000000e-01,
4.50000000e-01, 5.75000000e-01, 7.30000000e-01,
9.40000000e-01, 1.08500000e+00, 1.14000000e+00,
1.19500000e+00, 1.25500000e+00, 1.32000000e+00,
1.38500000e+00, 1.45000000e+00, 1.52500000e+00,
1.60000000e+00, 1.68000000e+00, 1.76500000e+00,
1.84500000e+00, 1.94000000e+00, 2.03500000e+00,
2.13500000e+00, 2.24000000e+00, 2.35000000e+00,
2.47500000e+00, 2.59000000e+00, 2.72500000e+00,
2.85000000e+00, 2.99000000e+00, 3.15000000e+00,
3.29500000e+00, 3.46500000e+00, 3.63000000e+00,
3.81500000e+00, 4.00500000e+00, 4.20500000e+00,
4.41500000e+00, 4.62500000e+00, 4.86500000e+00,
5.10500000e+00, 5.36000000e+00, 5.62000000e+00,
5.89500000e+00, 6.18000000e+00, 6.49000000e+00,
6.80500000e+00, 7.14000000e+00, 7.49500000e+00,
7.87000000e+00, 8.26000000e+00, 8.68500000e+00,
9.12500000e+00, 9.58500000e+00, 1.00800000e+01,
1.06000000e+01, 1.11500000e+01, 1.17300000e+01,
1.23400000e+01, 1.29850000e+01, 1.36650000e+01,
1.43700000e+01, 1.51150000e+01, 1.58900000e+01,
1.67000000e+01, 1.75450000e+01, 1.84200000e+01,
1.93150000e+01, 2.02200000e+01, 2.11600000e+01,
2.20800000e+01, 2.30300000e+01, 2.39950000e+01,
2.49600000e+01, 2.59400000e+01, 2.69150000e+01,
3.18400000e+01, 3.68050000e+01, 4.17550000e+01,
4.67450000e+01, 5.17150000e+01, 5.66950000e+01,
6.16750000e+01, 6.66600000e+01, 7.16350000e+01,
7.66150000e+01, 8.15900000e+01, 8.65700000e+01,
9.15500000e+01, 9.65300000e+01, 1.01520000e+02,
1.06490000e+02, 1.11470000e+02])
With some assistance, I got to this point.
import matplotlib.pyplot as plt
from scipy.interpolate import UnivariateSpline
y_spl = UnivariateSpline(x,y,s=0,k=4)
x_range = np.linspace(x[0],x[-1],1000)
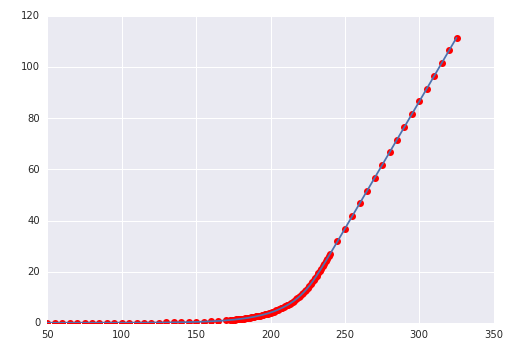
plt.plot(x,y,'ro',label = 'data')
plt.plot(x_range,y_spl(x_range))
This seems like a reasonably good fit. I want to take this polynomial and retrieve its results symbolically, because my numerical derivatives appear unnatural, even for this spline.
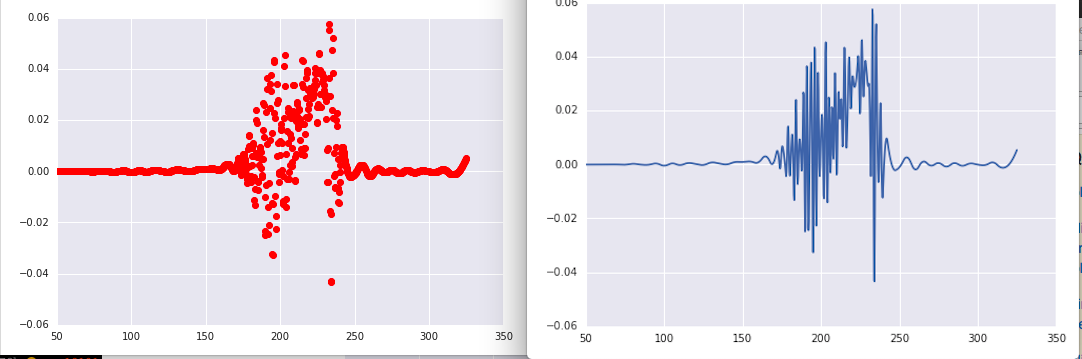
y_spl_2d = y_spl.derivative(n=2)
plt.plot(x_range,y_spl_2d(x_range))
I think even visually, this second derivative just doesn't cut it. The initial function appears pretty "smooth" in a math-sense.
What are my options from here? Can I retrieve the initial function symbolically and perform the derivative this way (analytically?). What other numerical procedures can I implement to smooth my second derivative? It doesn't appear to me at any point that my second derivative should be negative (and in a modeling sense with what I'm physically modeling, it should never be negative).
Can I retrieve the polynomial from the fit, or can I make numerical modifications to smooth my second derivative?
Edit: the goal is to retrieve a probability density function. I would also like to restrict this final derivative to be strictly positive and integrate to 1, but I think my problems are bigger than this right now. Those are secondary issues.
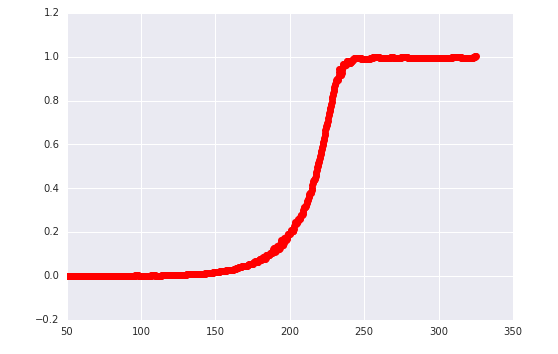
Here is the first derivative, which looks like a very fine-looking CDF, so I'm becoming more and more sure that my problems are numerical. Should I interpolate this result, as well, and differentiate? I'm sure these problems are very common - what are my next steps.
y_spl_2d = y_spl.derivative(n=1)
plt.plot(x_range,y_spl_2d(x_range), 'ro')