Central schemes like JST uses artificial dissipation for the stabilization. This modification is an artificial one. Does this additional term makes system inconsistent? Can we expect this term to be zero at the end of the simulation? This is in reference with incompressible flow schemes. In the following references they have used the pressure differences as a dissipation to continuity equations. Is it must to reach these terms to zero at the end of the simulation (steady)?
In principle for Artificial compressibility method lower values of norms of time derivative terms indicates the satisfaction of divergence of velocity. But in the following cases where artificial dissipation is added to continuity equation which will not go zero at the end of pseudo-transience. Will there also norms of time derivative terms indicates the satisfaction of divergence of velocity?
Reference 1(Link: http://heja.szif.hu/ANM/ANM-030110-A/anm030110a.pdf)
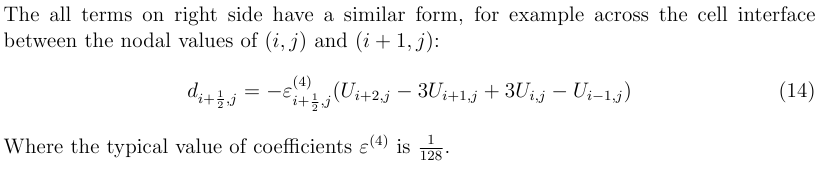
Reference 2(Link: http://www.sciencedirect.com/science/article/pii/S0021999199963155)
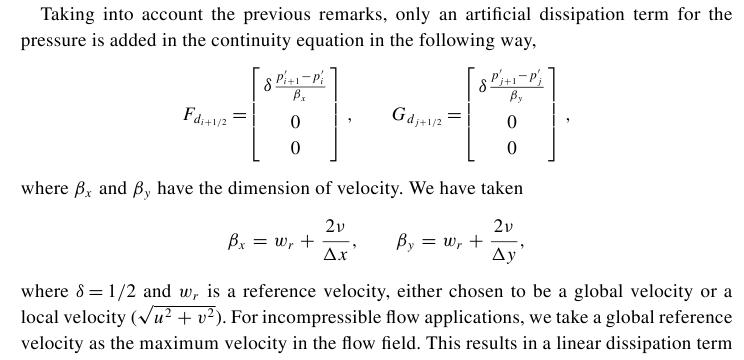
I have one more doubt. In principle for Artificial compressibility method lower values of norms of time derivative terms indicates the satisfaction of divergence of velocity. But in the above cases where artificial dissipation is added to continuity equation which will not go zero at the end of pseudo-transience. Will there also norms of time derivative terms indicates the satisfaction of divergence of velocity?