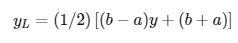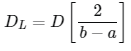EDIT:
As per requested here's the full description of my MMS test with results:
I) As an initial step I solved the linear Poisson's equation with the rescaled Chebyshev derivatives: $$ \nabla^2 u = S $$ where BCs are periodic in x and zero Dirichlet in y as $u(x,a)=0$ & $u(x,b)=0$.
For the simple above case the test I performed with the MMS is:
$$
u(x,y)=(y-a)(y-b)\sin{Ax}
$$
while $n(x,y)$ was set to 1, the above case works perfectly for me and I have no issue at all. The results of this case are the following:
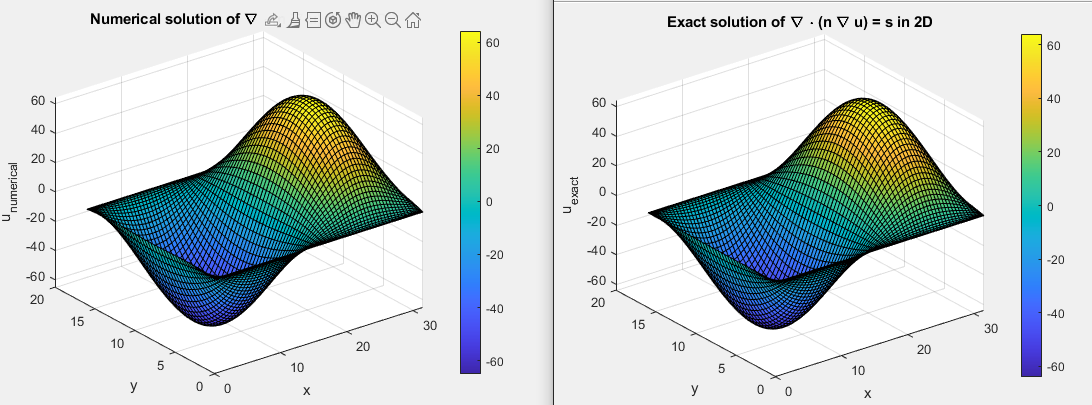
As you see above the numerical matches the exact solution for different domain lengths: $L_x=32; L_y=32,64,96,..$ and the adjusted code would be:
Nx = 4*16;
Ny = 4*16;
Lx =2*16;
kx = fftshift(-Nx/2:Nx/2-1); % wave number vector
dx = Lx/Nx;
% Use approximations for kx, and k^2. These come from Birdsall and Langdon
ksqu = (sin( kx * dx/2)/(dx/2)).^2 ;
kx = sin(kx * dx) / dx;
ksqu4inv = ksqu;
ksqu4inv(abs(ksqu4inv)<1e-14) =1; %helps with error: matrix ill scaled because of 0s
xi_x = 2*pi/Lx;
xi = (0:Nx-1)/Nx*2*pi;
x = xi/xi_x;
ylow = 0; %a
yupp =16; %b
Ly = (yupp-ylow);
eta_ygl = 2/Ly;
[D,etagl] = cheb(Ny);
% rescaling my Chebyshev Differentiation Matrices to an arbitrary domain [a,b] by performing a change of variables as the following:
ygl = (1/2)*((yupp-ylow)*etagl + (yupp+ylow));
D = D*eta_ygl;
D2 = D*D;
[X,Y] = meshgrid(x,ygl);
Igl = speye(Ny+1);
%ZNy represents the operation of setting the boundary values of y component
%to zero:
ZNy = diag([0 ones(1,Ny-1) 0]); %diag([0 ones(1,Ny-1) 0]);
div_y_act_on_grad_y = D2* ZNy;
%ICs
A = 2*pi / Lx;
u = (Y-ylow) .* (Y-yupp) .* sin( (A) * X);
uh = fft(u,[],2);
n = ones(size(u)); %set to 1
invnek = fft(1./n,[],2);
nh = fft(n,[],2);
dnhdxk = (kx*1i*xi_x).*nh;
dnhdyk =D * nh;
%Linear Exact source
LExactSource = (4*pi^2*(ylow-Y).*(Y-yupp).*sin(A*X))/Lx^2 + 2*sin(A*X);
oldSol = ones(size(u));
oldSolk = fft(oldSol ,[],2);
err_max =1e-4;
max_iter = 500;
Sourcek = fft(LExactSource ,[],2);
for iterations = 1:max_iter
oldSolMax = max(max(abs(oldSolk)));
dudxk = (kx*1i*xi_x) .*oldSolk;
%product:
gradNgradUx = aapx(dnhdxk,dudxk);
dudyk = (D) *oldSolk ;
gradNgradUy = aapx(dnhdyk,dudyk);
%RHS of PDE
RHSk = Sourcek - (gradNgradUx + gradNgradUy);
Stilde = aapx(invnek,RHSk);
for m = 1:length(kx)
L = -Igl * (ksqu4inv(m))*xi_x^2+ div_y_act_on_grad_y;
newSolk(:,m) = L\(Stilde(:,m));
end
%enforce BCs
newSolk=[zeros(1,Nx); newSolk(2:Ny,:); zeros(1,Nx)];
newSolMax = max(max(abs(newSolk)));
if newSolMax < err_max
it_error = err_max /2;
else
it_error = abs( newSolMax - oldSolMax ) / newSolMax ;
end
if it_error < err_max
break;
end
oldSolk = newSolk;
end
%plot numerical solution vs exact
newSol= real(ifft(newSolk,[],2));
figure
surf(X, Y, newSol);
colorbar;
figure
surf(X, Y, u);
colorbar;
II) However, when I try to do the same for the nonlinear Poisson's equation with following expression: $$ \nabla \cdot (n\nabla u) = S $$ or can be expanded as: $n \nabla^2 u + \nabla u \cdot \nabla n = S$
Then, using the MMS I chose $u(x,y)$ and $n(x,y)$ similarly as the first case:
$$
u(x,y)=(y-a)(y-b)\sin{Ax}
$$
$$
n(x,y)=\left(\frac{2}{b-a}\right)\left[y-\left(\frac{a+b}{2}\right) \right]^2\sin{3Ax}+10
$$
For the above nonlinear case with $L_x=32, L_y=16$ I get the following results:
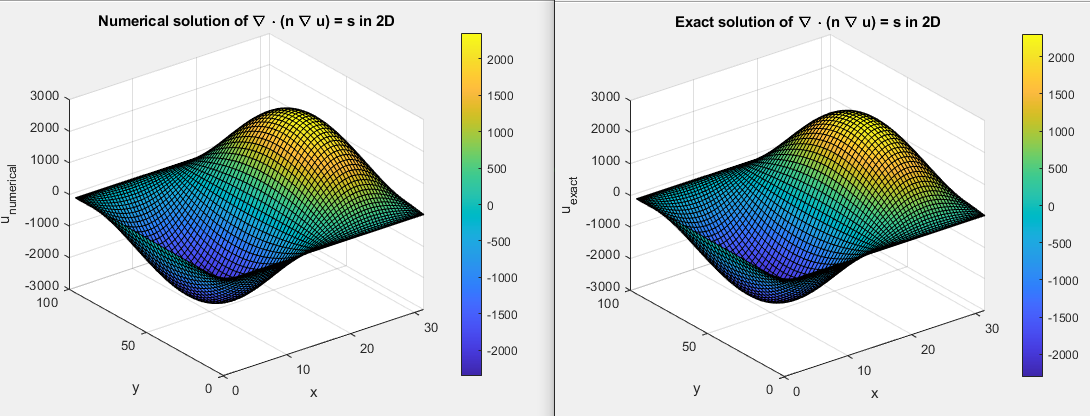
However, if I change my domain length along y$L_x=32L_y=32$ this results in the solution blowing up as the following:
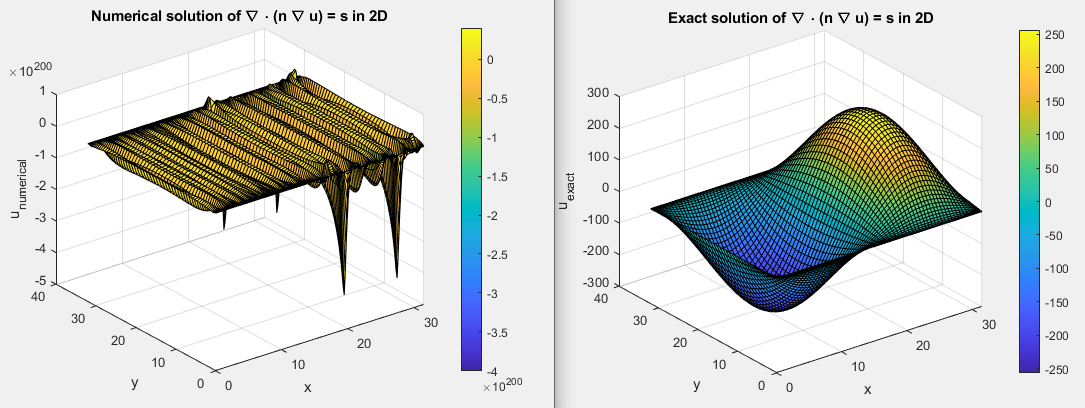So, basically for the nonlinear case the code works for $L_y$ from 0 to 16 then blows up! I don't seem to see a pattern to begin to understand where the issue maybe. I have done an order of accuracy test where $L_x=L_y=16$ (case where solution does NOT blow up) and it gives me expected order of accuracy so any insight here would be helpful.
