Order of element vs Degrees of freedom of the element

Question

I have read that the order of the element is the order of the polynomial used to approximate/represent the field variable in that element. If we consider a one-dimensional, 2 degrees of freedom element (with 2 nodes) the polynomial would be a linear polynomial and if this element happens to have an interior node (so totally 3 nodes, and 3 DOF), then the polynomial would be a quadratic polynomial.

I have also read that the degree of the polynomial we choose also depends on the degrees of freedom of the element. So, if we consider a 4 DOF element, then the polynomial would be cubic polynomial. But even though we use a cubic polynomial the element is still a linear element.

Here is my confusion: Would a quadratic polynomial still be able to represent a one dimensional, 3 noded elements (i.e. with 1 interior node) with 2 DOF at each node (so a 6 DOF element)? I am asking this because usually a 1 dimension 3 noded element would be called a quadratic element...but the textbooks always assume a single degree of freedom at each node. Would this element (with 3 nodes) still be called a 'quadratic-element' if the number of degrees of freedom per node is increased to 2?

This is what I have been referring to study FEM along with notes from NPTEL:

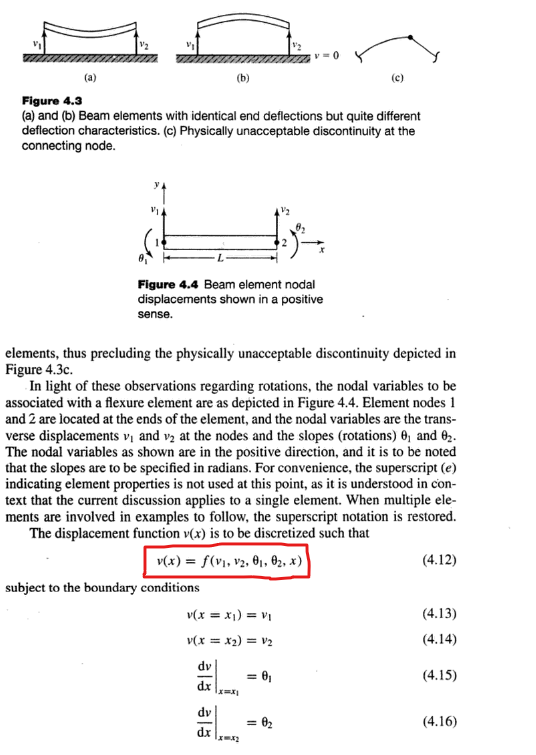
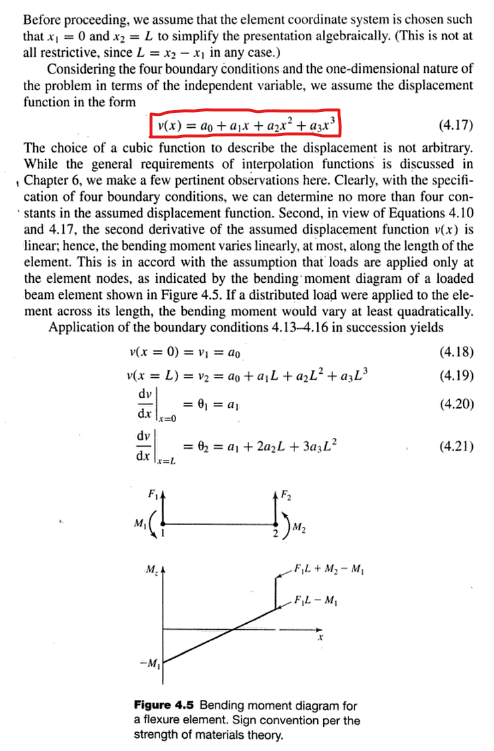
The beam element has 4 DOF, given by transverse displacement and its slope.

The displacement field variable is approximated by a cubic polynomial. So is the beam element a cubic element?

Updated question:

After studying about Hermite interpolation I know that it not only ensures continuity of the given DOF (let's take x-displacement) but also the continuity of its derivative till a given order of the derivative. So applying this understanding to my question I know come to understand why this is important as the rotation DOF is the derivative of the displacement DOF.

Now knowing this I would like to refine my previous question with which I think should clear this up for me.

Let's take 2 cases. Each case comparing a 1D 2-noded element and a 1D 3-noded element (ie with 1 interior node):

Case 1: Each node has 2 DoF for the 2 elements. Let them be x-displacement and rotation. In this case, I know we use hermite shape function. So the approximation function for x-displacement will be given as

u(x) = sum(ui * Ni) + sum(u'i * Mi)

Where u(x) is the approx function for displacement, ui is the nodal displacement, Ni shape function for nodal displacement, u'i is the derivative of nodal displacement at the node, and Mi shape function for derivative of displacement.

a) What about the value for rotation DOF? Will, there be an approximation function for the rotation DOF as well?

b) Taking the 2 noded element, I know that this element has a total of 4 DOF. For this will we be using a cubic polynomial? Also, what will the order of the element be? (Is it a cubic element even though it has just 2 nodes?)

c) Taking the 3 noded element, I know that this element has a total of 6 DOF. For this will we be using a 5th order polynomial? Also, what will the order of the element be? ( Is it a 5th order element or will it be a quadratic element as it has 3 nodes?

Case 2: Lets again consider that each node has 2 DOF. But this time let the DoF be x-displacement and temperature (or x-displacement and y-displacement).

a) In this case would the approximation function for x-displacement be :

u(x) = sum(ui * Ni) + sum(Ti * Mi) where Ti is the nodal temperature and Mi is the shape function for temprerature DoF.

b) What about Temperature? Would there be approximation function for temperature DoF too? (some thing like T(x) = sum(Ti * Si) + sum(ui * Qi) ??

c) What will the order of the element be for the 2 noded element and the 3 noded element?

Please help me out with this. Thank you

Answer 1

A quadratic polynomial wouldn't always be able to do that. It depends on what the DOFs represent. Often a DOF corresponds to the value of the basis function at the node point, but it doesn't have to. We could for instance have two colocated DOFs at each node where one corresponds to the basis function value and the other its derivative. This would generally require a 5th order polynomial to satisfy.

Here's a simpler 2-node four degree of freedom example. Using the following basis functions, $$ \phi_1(x) = -\frac{1}{2}(x-1) \\ \phi_2(x) = \frac{1}{4}(x+1)(x-1)^2 \\ \phi_3(x) = -\frac{1}{4}(x+1)^2(x-1) \\ \phi_4(x) = \frac{1}{2}(x+1), $$ the degrees of freedom associated with basis functions 1 and 4 correspond to the value at nodes $x=-1$ and $x=1$, whereas the degrees of freedom for basis functions 2 and 3 represent their derivatives because they have unit derivatives at the nodes.

If the solution to our problem requires a function such that $$f(-1)=0, f'(-1)=1, f(1)=0, f'(1)=1,$$ we would need a cubic, not linear polynomial.

Answer 2

The correct way to frame your question is to consider the equation you are going to solve.

For simplicity consider the pure 1D problem, where your domain is in $\mathbb{R}^1$, e.g. $0 < x < l$. (Restrict the analysis to 1D elements which lie on the $x$ axis, not considering the case of 1D elements in the $\mathbb{R}^2$ plane or in the $\mathbb{R}^3$ space.)

Define

• $E$, Young Modulus,
• $A$ area,
• $I$ second area moment,
• $f_x, f_y$ force per unit of length in $x$ and $y$ directions, respectively
• $u(x)$ axial displacement, along $x$ direction
• $v(x)$ transverse displacement, along $y$ direction.

You can have two different problems.

• Axial displacements $u(x)$ governed by the equation $$ - EA \frac{\mathrm d^2}{\mathrm d x^2} u(x) = f_x(x), \qquad \text{and relevant b.c.} $$ In this case you have no bending, but only axial deformation.

• Transverse displacement $v(x)$ governed by the equation $$ EI \frac{\mathrm d^4}{\mathrm d x^4} v(x) = f_y(x), \qquad \text{and relevant b.c.} $$ Now you have only bending (flexure).

Now it is clear that for $u(x)$ (second order diff. equation) and $v(x)$ (fourth order diff. equation) you have different regularity requirements. More precisely for a weak formulation you need $u \in H^1$ but $v \in H^2$, which roughly translates to $C^0$ continuity for $u$ and $C^1$ for $v$. For this reason for $u$ one has 1 DOF at nodes, while for $v$ on has 2 DOF ($v$ and $\frac{\mathrm d v}{\mathrm d x}$) to guarantee the correct regularity of the solution.

2 node beam elements usually combine the above two problems (which are indeed orthogonal in the energy norm), for a total of 3 DOF per node, which are also retained in a 2D formulation (straight element in $x,y$ plane).

In conclusion: the order of the governing differential equation determine the regularity requirements, and thus the type and order of the basis functions.