0
$\begingroup$

I am modelling a problem that is "driven", not by typical boundary conditions but, by contractions in its interior.

In a finite element analysis, I can specify the new lengths (not displacement vectors) of a set, $\mathscr{S}$, of element edges. I do not know the final positions of the vertexes that make up edges in $\mathscr{S}$, just the lengths between the vertexes in the set.

Is there a finite element technique for dealing with such a problem?

Clarification

The problem is characterized by clearly defined internal structures that shrink by a known amount. The geometry/definition of these internal structures can be identified in the discretization of the domain, which also has fixed boundary conditions.

The geometry of the the internal structure does not have an analytical description and the Physics that describes it's contraction is not relevant. Just that the structure undergoes a known reduction in size.

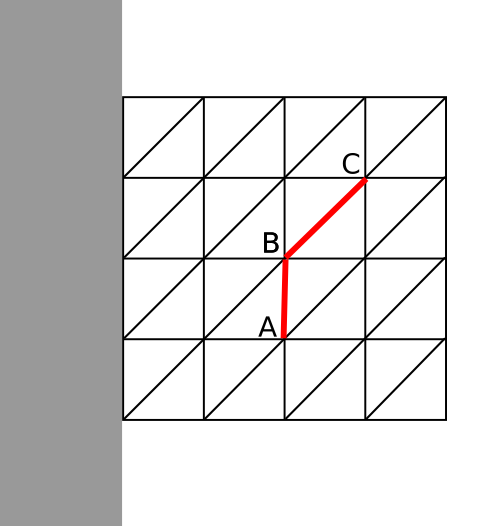
enter image description here

For example, what would be the nodal point displacements if the lengths of the edges AB and BC, in the above figure, were both halved?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ You're starting at the wrong end, with the mesh. Write down what the partial differential equation is that you're trying to solve, and the let's look at the discretization you can come up with. $\endgroup$
    – Wolfgang Bangerth
    Commented Jan 9, 2021 at 4:45
  • 1
    $\begingroup$ If this question is interpreted in the context of elasticity, it kind of makes sense what you are asking. However, although in a discrete setting it is possible to, e.g., penalize the distance between two nodes, it may not as such correspond to a well posed boundary value problem because defining point constraints can lead to divergent numerical methods. Furthermore, I think this will make a linear problem nonlinear but did not check the details at all. You might want to add more specifics, e.g., a simplified example. As Wolfgang said, you also want to confirm that we're talking elasticity. $\endgroup$
    – knl
    Commented Jan 9, 2021 at 7:53
  • $\begingroup$ @knl Thanks for your feedback. I am thinking in the context of hyperelasticity. Intuitively, I expected the problem to be ill-posed and non-linear but hope(d) there would be a way to regularize it. The PDEs are the same ones encountered in solid deformation. At the moment I am trying to find out how best to solve the problem. Perhaps someone has published anything on a similar problem. $\endgroup$
    – Olumide
    Commented Jan 10, 2021 at 23:47

1 Answer 1

Reset to default
3
$\begingroup$

This can be solved as follows.

If $L_0$ is the initial distance between the two nodes you want to displace, $L$ is the distance between the two nodes in the displaced body, and $d$ is the amount of length change you want to define, the following constraint relation can be defined

$$ G=L-L_0-d=0 $$ Note that $L$ is a nonlinear function of the nodal displacements, $u$. A common way of enforcing this constraint is by defining a Lagrange multiplier, $\lambda$. In the general case, where the structural behavior is nonlinear (due, e.g. to geometric or material nonlinearities), we have the following system of $N+1$ nonlinear equations

\begin{eqnarray} f^{int} + \lambda\frac{\partial G}{\partial u} &=& 0 \nonumber \\ L&=&L_0 + d \nonumber \end{eqnarray} where $f^{int}$ is the vector of internal forces at the nodes.

If the finite element equations (excluding the constraint equation) are linear, $f^{int}$ can be replaced with $Ku$ where $K$ is the usual global stiffness matrix and $u$ is the vector of nodal displacements of length $N$. These equations can be solved by standard approaches, e.g. Newton-Raphson.

Improve this answer
$\endgroup$
10
  • $\begingroup$ Thanks for your reply. (I took time off to study non-linear continuum mechanics & FEA.) Is it a problem that $L(u)$ is a square root? Surely this will make it impossible to create a linear system. In that case, why not $G = (L_0 - d)^2 - L(u)^2$. I have used $L_0 - d$ because the displacement $d$ is a contraction. $\endgroup$
    – Olumide
    Commented Feb 27, 2021 at 5:46
  • $\begingroup$ If the displacements are small, you can certainly linearize the constraint equation. $\endgroup$
    – Bill Greene
    Commented Feb 27, 2021 at 10:00
  • $\begingroup$ The deformations are not small and the material is hyperelastic. That's why I've been studying nonlinear continuum mechanics/FEA. (I am currently working my way through Klaus-Jürgen Bathe's excellent course.) $\endgroup$
    – Olumide
    Commented Feb 27, 2021 at 12:39
  • $\begingroup$ Why $Ku + \lambda \frac{\partial G}{\partial u}$ and not $Ku + \lambda G$? $\endgroup$
    – Olumide
    Commented May 12, 2021 at 13:42
  • $\begingroup$ Thanks @bill-greene. I am very grateful for your help, it took me a while to fully appreciate your answer I finally got there. My first implementation on a purely elastic material seems to work well. I plan to try with larger scale problems before moving to a hyper-elastic material. $\endgroup$
    – Olumide
    Commented May 26, 2021 at 17:21

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.