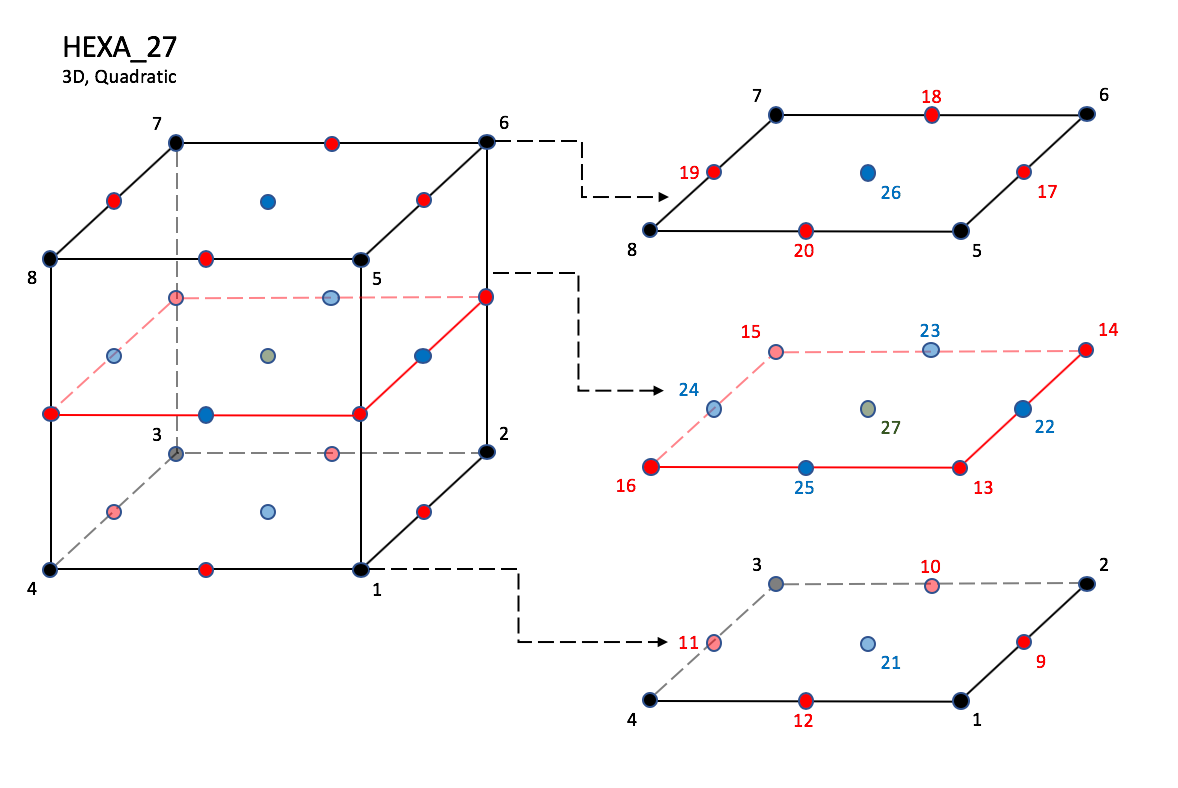
Given 27 $(x,y,z)$ coordinates in 3D space which describe a generally curved quadratic hexahedron, which correspond to the HEXA_27 reference element figure with planar faces in $(\xi, \eta, \zeta)$ coordinates. Nodes constructed on the interior by tensor-products of Gauss-Legendre nodes need associated metric terms at those nodes, $\partial(x,y,z)/\partial(\xi,\eta,\zeta)$.
How does one compute those metric terms given those 3D points as input?
Let's assume you have a way of computing the 27 tri-quadratic shape functions associated with the 27 points (either by tabulating them as polynomials, or by computing them as interpolating Lagrange polynomials satisfying $\varphi_i(\vec \xi_j) = \delta_{ij}$), then the mapping from the reference cell is provided by $$ \vec x(\vec\xi) = \sum_{i=1}^{27} \vec v_i \varphi_i(\vec\xi) $$ where $\vec v_i$ are the 27 node positions in real space.
You use this mapping applied to the reference location of quadrature points to determine their real-space location. The Jacobian matrix of this mapping is given by $$ J(\vec\xi) = \sum_{i=1}^{27} \vec v_i \otimes \nabla\varphi_i(\vec\xi) $$ (or its transpose, depending on how exactly you define the Jacobian).
