I have two algebraic equations I am trying to solve in Maple. They are:
$14\,{a}^{26}{b}^{2}-91\,{a}^{24}{b}^{4}-364\,{a}^{22}{b}^{6}-1001\,{a} ^{20}{b}^{8}-2002\,{a}^{18}{b}^{10}-3003\,{a}^{16}{b}^{12}-3432\,{a}^{ 14}{b}^{14}-3003\,{a}^{12}{b}^{16}-2002\,{a}^{10}{b}^{18}-1001\,{a}^{8 }{b}^{20}-364\,{a}^{6}{b}^{22}-91\,{a}^{4}{b}^{24}-14\,{a}^{2}{b}^{26} -{b}^{28}-36\,{a}^{26}-396\,{a}^{24}{b}^{2}-1944\,{a}^{22}{b}^{4}-5544 \,{a}^{20}{b}^{6}-9900\,{a}^{18}{b}^{8}-10692\,{a}^{16}{b}^{10}-4752\, {a}^{14}{b}^{12}+4752\,{a}^{12}{b}^{14}+10692\,{a}^{10}{b}^{16}+9900\, {a}^{8}{b}^{18}+5544\,{a}^{6}{b}^{20}+1944\,{a}^{4}{b}^{22}+396\,{a}^{ 2}{b}^{24}+36\,{b}^{26}+1872\,{a}^{24}+16576\,{a}^{22}{b}^{2}+64672\,{ a}^{20}{b}^{4}+146880\,{a}^{18}{b}^{6}+220080\,{a}^{16}{b}^{8}+246144 \,{a}^{14}{b}^{10}+245952\,{a}^{12}{b}^{12}+246144\,{a}^{10}{b}^{14}+ 220080\,{a}^{8}{b}^{16}+146880\,{a}^{6}{b}^{18}+64672\,{a}^{4}{b}^{20} +16576\,{a}^{2}{b}^{22}+1872\,{b}^{24}-25792\,{a}^{22}-173248\,{a}^{20 }{b}^{2}-490560\,{a}^{18}{b}^{4}-756800\,{a}^{16}{b}^{6}-672640\,{a}^{ 14}{b}^{8}-258944\,{a}^{12}{b}^{10}+258944\,{a}^{10}{b}^{12}+672640\,{ a}^{8}{b}^{14}+756800\,{a}^{6}{b}^{16}+490560\,{a}^{4}{b}^{18}+173248 \,{a}^{2}{b}^{20}+25792\,{b}^{22}+198144\,{a}^{20}+1956864\,{a}^{18}{b }^{2}+6753792\,{a}^{16}{b}^{4}+11292672\,{a}^{14}{b}^{6}+10742784\,{a} ^{12}{b}^{8}+8890368\,{a}^{10}{b}^{10}+10742784\,{a}^{8}{b}^{12}+ 11292672\,{a}^{6}{b}^{14}+6753792\,{a}^{4}{b}^{16}+1956864\,{a}^{2}{b} ^{18}+198144\,{b}^{20}-731136\,{a}^{18}-12589056\,{a}^{16}{b}^{2}- 37822464\,{a}^{14}{b}^{4}-45244416\,{a}^{12}{b}^{6}-19279872\,{a}^{10} {b}^{8}+19279872\,{a}^{8}{b}^{10}+45244416\,{a}^{6}{b}^{12}+37822464\, {a}^{4}{b}^{14}+12589056\,{a}^{2}{b}^{16}+731136\,{b}^{18}-9461760\,{a }^{16}-30867456\,{a}^{14}{b}^{2}+287145984\,{a}^{12}{b}^{4}+369033216 \,{a}^{10}{b}^{6}+120963072\,{a}^{8}{b}^{8}+369033216\,{a}^{6}{b}^{10} +287145984\,{a}^{4}{b}^{12}-30867456\,{a}^{2}{b}^{14}-9461760\,{b}^{16 }+85622784\,{a}^{14}+350257152\,{a}^{12}{b}^{2}-255688704\,{a}^{10}{b} ^{4}-3238232064\,{a}^{8}{b}^{6}+3238232064\,{a}^{6}{b}^{8}+255688704\, {a}^{4}{b}^{10}-350257152\,{a}^{2}{b}^{12}-85622784\,{b}^{14}+ 137035776\,{a}^{12}-5009965056\,{a}^{10}{b}^{2}-3606773760\,{a}^{8}{b} ^{4}+24823726080\,{a}^{6}{b}^{6}-3606773760\,{a}^{4}{b}^{8}-5009965056 \,{a}^{2}{b}^{10}+137035776\,{b}^{12}-1408499712\,{a}^{10}-1054605312 \,{a}^{8}{b}^{2}+81891164160\,{a}^{6}{b}^{4}-81891164160\,{a}^{4}{b}^{ 6}+1054605312\,{a}^{2}{b}^{8}+1408499712\,{b}^{10}+1443889152\,{a}^{8} +56962842624\,{a}^{6}{b}^{2}-171624628224\,{a}^{4}{b}^{4}+56962842624 \,{a}^{2}{b}^{6}+1443889152\,{b}^{8}+13929283584\,{a}^{6}-97504985088 \,{a}^{4}{b}^{2}+97504985088\,{a}^{2}{b}^{4}-13929283584\,{b}^{6}=0$
and
$75\,{a}^{44}+1250\,{a}^{42}{b}^{2}+9325\,{a}^{40}{b}^{4}+39500\,{a}^{ 38}{b}^{6}+92625\,{a}^{36}{b}^{8}+37050\,{a}^{34}{b}^{10}-605625\,{a}^ {32}{b}^{12}-2713200\,{a}^{30}{b}^{14}-7025250\,{a}^{28}{b}^{16}- 13081500\,{a}^{26}{b}^{18}-18685550\,{a}^{24}{b}^{20}-20995000\,{a}^{ 22}{b}^{22}-18685550\,{a}^{20}{b}^{24}-13081500\,{a}^{18}{b}^{26}- 7025250\,{a}^{16}{b}^{28}-2713200\,{a}^{14}{b}^{30}-605625\,{a}^{12}{b }^{32}+37050\,{a}^{10}{b}^{34}+92625\,{a}^{8}{b}^{36}+39500\,{a}^{6}{b }^{38}+9325\,{a}^{4}{b}^{40}+1250\,{a}^{2}{b}^{42}+75\,{b}^{44}-6360\, {a}^{42}-124360\,{a}^{40}{b}^{2}-1141040\,{a}^{38}{b}^{4}-6517200\,{a} ^{36}{b}^{6}-25897560\,{a}^{34}{b}^{8}-75690120\,{a}^{32}{b}^{10}- 167296320\,{a}^{30}{b}^{12}-281699520\,{a}^{28}{b}^{14}-354817200\,{a} ^{26}{b}^{16}-309064080\,{a}^{24}{b}^{18}-123936800\,{a}^{22}{b}^{20}+ 123936800\,{a}^{20}{b}^{22}+309064080\,{a}^{18}{b}^{24}+354817200\,{a} ^{16}{b}^{26}+281699520\,{a}^{14}{b}^{28}+167296320\,{a}^{12}{b}^{30}+ 75690120\,{a}^{10}{b}^{32}+25897560\,{a}^{8}{b}^{34}+6517200\,{a}^{6}{ b}^{36}+1141040\,{a}^{4}{b}^{38}+124360\,{a}^{2}{b}^{40}+6360\,{b}^{42 }-286560\,{a}^{40}+1299840\,{a}^{38}{b}^{2}+50378944\,{a}^{36}{b}^{4}+ 401336704\,{a}^{34}{b}^{6}+1740940320\,{a}^{32}{b}^{8}+4901465600\,{a} ^{30}{b}^{10}+9580140800\,{a}^{28}{b}^{12}+13378708992\,{a}^{26}{b}^{ 14}+13616978752\,{a}^{24}{b}^{16}+10903809280\,{a}^{22}{b}^{18}+ 9196936320\,{a}^{20}{b}^{20}+10903809280\,{a}^{18}{b}^{22}+13616978752 \,{a}^{16}{b}^{24}+13378708992\,{a}^{14}{b}^{26}+9580140800\,{a}^{12}{ b}^{28}+4901465600\,{a}^{10}{b}^{30}+1740940320\,{a}^{8}{b}^{32}+ 401336704\,{a}^{6}{b}^{34}+50378944\,{a}^{4}{b}^{36}+1299840\,{a}^{2}{ b}^{38}-286560\,{b}^{40}+372661248\,{a}^{36}{b}^{2}-707820544\,{a}^{34 }{b}^{4}-13983124480\,{a}^{32}{b}^{6}-64604672000\,{a}^{30}{b}^{8}- 171060842496\,{a}^{28}{b}^{10}-301423489024\,{a}^{26}{b}^{12}- 369866092544\,{a}^{24}{b}^{14}-305887582208\,{a}^{22}{b}^{16}- 118022141952\,{a}^{20}{b}^{18}+118022141952\,{a}^{18}{b}^{20}+ 305887582208\,{a}^{16}{b}^{22}+369866092544\,{a}^{14}{b}^{24}+ 301423489024\,{a}^{12}{b}^{26}+171060842496\,{a}^{10}{b}^{28}+ 64604672000\,{a}^{8}{b}^{30}+13983124480\,{a}^{6}{b}^{32}+707820544\,{ a}^{4}{b}^{34}-372661248\,{a}^{2}{b}^{36}-64023552\,{b}^{38}- 4020256512\,{a}^{36}-25280404992\,{a}^{34}{b}^{2}-17689454336\,{a}^{32 }{b}^{4}+327596421120\,{a}^{30}{b}^{6}+1568612611072\,{a}^{28}{b}^{8}+ 3802104150016\,{a}^{26}{b}^{10}+5990533923840\,{a}^{24}{b}^{12}+ 6790271389696\,{a}^{22}{b}^{14}+6232580648448\,{a}^{20}{b}^{16}+ 5750651833344\,{a}^{18}{b}^{18}+6232580648448\,{a}^{16}{b}^{20}+ 6790271389696\,{a}^{14}{b}^{22}+5990533923840\,{a}^{12}{b}^{24}+ 3802104150016\,{a}^{10}{b}^{26}+1568612611072\,{a}^{8}{b}^{28}+ 327596421120\,{a}^{6}{b}^{30}-17689454336\,{a}^{4}{b}^{32}-25280404992 \,{a}^{2}{b}^{34}-4020256512\,{b}^{36}+141841483776\,{a}^{34}+ 812651771904\,{a}^{32}{b}^{2}+844669190144\,{a}^{30}{b}^{4}- 5879063412736\,{a}^{28}{b}^{6}-27045676376064\,{a}^{26}{b}^{8}- 58879853699072\,{a}^{24}{b}^{10}-81000773943296\,{a}^{22}{b}^{12}- 71903915409408\,{a}^{20}{b}^{14}-28790241103872\,{a}^{18}{b}^{16}+ 28790241103872\,{a}^{16}{b}^{18}+71903915409408\,{a}^{14}{b}^{20}+ 81000773943296\,{a}^{12}{b}^{22}+58879853699072\,{a}^{10}{b}^{24}+ 27045676376064\,{a}^{8}{b}^{26}+5879063412736\,{a}^{6}{b}^{28}- 844669190144\,{a}^{4}{b}^{30}-812651771904\,{a}^{2}{b}^{32}- 141841483776\,{b}^{34}-3238367662080\,{a}^{32}-15304336146432\,{a}^{30 }{b}^{2}-9340373663744\,{a}^{28}{b}^{4}+95140684300288\,{a}^{26}{b}^{6 }+333410977595392\,{a}^{24}{b}^{8}+614533778046976\,{a}^{22}{b}^{10}+ 845198215839744\,{a}^{20}{b}^{12}+1012450883862528\,{a}^{18}{b}^{14}+ 1081581115908096\,{a}^{16}{b}^{16}+1012450883862528\,{a}^{14}{b}^{18}+ 845198215839744\,{a}^{12}{b}^{20}+614533778046976\,{a}^{10}{b}^{22}+ 333410977595392\,{a}^{8}{b}^{24}+95140684300288\,{a}^{6}{b}^{26}- 9340373663744\,{a}^{4}{b}^{28}-15304336146432\,{a}^{2}{b}^{30}- 3238367662080\,{b}^{32}+50490836582400\,{a}^{30}+176086600974336\,{a}^ {28}{b}^{2}-152306959187968\,{a}^{26}{b}^{4}-1684710750093312\,{a}^{24 }{b}^{6}-3454899096584192\,{a}^{22}{b}^{8}-3298794461921280\,{a}^{20}{ b}^{10}-1445416723218432\,{a}^{18}{b}^{12}-194713331367936\,{a}^{16}{b }^{14}+194713331367936\,{a}^{14}{b}^{16}+1445416723218432\,{a}^{12}{b} ^{18}+3298794461921280\,{a}^{10}{b}^{20}+3454899096584192\,{a}^{8}{b}^ {22}+1684710750093312\,{a}^{6}{b}^{24}+152306959187968\,{a}^{4}{b}^{26 }-176086600974336\,{a}^{2}{b}^{28}-50490836582400\,{b}^{30}- 534649494896640\,{a}^{28}-1210295967940608\,{a}^{26}{b}^{2}+ 5724614944423936\,{a}^{24}{b}^{4}+26380886835724288\,{a}^{22}{b}^{6}+ 42048454899204096\,{a}^{20}{b}^{8}+30388730092978176\,{a}^{18}{b}^{10} +4724812444139520\,{a}^{16}{b}^{12}-7192176335781888\,{a}^{14}{b}^{14} +4724812444139520\,{a}^{12}{b}^{16}+30388730092978176\,{a}^{10}{b}^{18 }+42048454899204096\,{a}^{8}{b}^{20}+26380886835724288\,{a}^{6}{b}^{22 }+5724614944423936\,{a}^{4}{b}^{24}-1210295967940608\,{a}^{2}{b}^{26}- 534649494896640\,{b}^{28}+3286873284280320\,{a}^{26}+2713917651943424 \,{a}^{24}{b}^{2}-77284074436165632\,{a}^{22}{b}^{4}- 270542370073739264\,{a}^{20}{b}^{6}-349879051057889280\,{a}^{18}{b}^{8 }-213063378711085056\,{a}^{16}{b}^{10}-57015578923106304\,{a}^{14}{b}^ {12}+57015578923106304\,{a}^{12}{b}^{14}+213063378711085056\,{a}^{10}{ b}^{16}+349879051057889280\,{a}^{8}{b}^{18}+270542370073739264\,{a}^{6 }{b}^{20}+77284074436165632\,{a}^{4}{b}^{22}-2713917651943424\,{a}^{2} {b}^{24}-3286873284280320\,{b}^{26}+213111700193280\,{a}^{24}+ 70908012205703168\,{a}^{22}{b}^{2}+694283115155161088\,{a}^{20}{b}^{4} +2132555668861747200\,{a}^{18}{b}^{6}+1859501969611161600\,{a}^{16}{b} ^{8}+84575734147842048\,{a}^{14}{b}^{10}-75928100231184384\,{a}^{12}{b }^{12}+84575734147842048\,{a}^{10}{b}^{14}+1859501969611161600\,{a}^{8 }{b}^{16}+2132555668861747200\,{a}^{6}{b}^{18}+694283115155161088\,{a} ^{4}{b}^{20}+70908012205703168\,{a}^{2}{b}^{22}+213111700193280\,{b}^{ 24}-197112346768834560\,{a}^{22}-1313568123575599104\,{a}^{20}{b}^{2}- 4826743947927748608\,{a}^{18}{b}^{4}-15507264587736023040\,{a}^{16}{b} ^{6}-13320253101815365632\,{a}^{14}{b}^{8}+6228544173411139584\,{a}^{ 12}{b}^{10}-6228544173411139584\,{a}^{10}{b}^{12}+13320253101815365632 \,{a}^{8}{b}^{14}+15507264587736023040\,{a}^{6}{b}^{16}+ 4826743947927748608\,{a}^{4}{b}^{18}+1313568123575599104\,{a}^{2}{b}^{ 20}+197112346768834560\,{b}^{22}+1396772423168163840\,{a}^{20}+ 11608333269149417472\,{a}^{18}{b}^{2}+24257517869881884672\,{a}^{16}{b }^{4}+70758399650983575552\,{a}^{14}{b}^{6}+96421712044744507392\,{a}^ {12}{b}^{8}-128968645422643937280\,{a}^{10}{b}^{10}+ 96421712044744507392\,{a}^{8}{b}^{12}+70758399650983575552\,{a}^{6}{b} ^{14}+24257517869881884672\,{a}^{4}{b}^{16}+11608333269149417472\,{a}^ {2}{b}^{18}+1396772423168163840\,{b}^{20}-433185960612593664\,{a}^{18} -55461219976548974592\,{a}^{16}{b}^{2}-157656713576520351744\,{a}^{14} {b}^{4}-153912151266903982080\,{a}^{12}{b}^{6}-760803075000404803584\, {a}^{10}{b}^{8}+760803075000404803584\,{a}^{8}{b}^{10}+ 153912151266903982080\,{a}^{6}{b}^{12}+157656713576520351744\,{a}^{4}{ b}^{14}+55461219976548974592\,{a}^{2}{b}^{16}+433185960612593664\,{b}^ {18}-37561374368266715136\,{a}^{16}-32837068847629467648\,{a}^{14}{b}^ {2}+1163976553479951876096\,{a}^{12}{b}^{4}+42543947772724248576\,{a}^ {10}{b}^{6}+1705420374725986615296\,{a}^{8}{b}^{8}+ 42543947772724248576\,{a}^{6}{b}^{10}+1163976553479951876096\,{a}^{4}{ b}^{12}-32837068847629467648\,{a}^{2}{b}^{14}-37561374368266715136\,{b }^{16}+145123568542084497408\,{a}^{14}+2214786761572522917888\,{a}^{12 }{b}^{2}-2941686581914050232320\,{a}^{10}{b}^{4}- 10669449418591224987648\,{a}^{8}{b}^{6}+10669449418591224987648\,{a}^{ 6}{b}^{8}+2941686581914050232320\,{a}^{4}{b}^{10}- 2214786761572522917888\,{a}^{2}{b}^{12}-145123568542084497408\,{b}^{14 }+249314329329842257920\,{a}^{12}-7902762540911007105024\,{a}^{10}{b}^ {2}-25076225098692949966848\,{a}^{8}{b}^{4}+83375468150158025293824\,{ a}^{6}{b}^{6}-25076225098692949966848\,{a}^{4}{b}^{8}- 7902762540911007105024\,{a}^{2}{b}^{10}+249314329329842257920\,{b}^{12 }-2801419437596265676800\,{a}^{10}-4512413307974543474688\,{a}^{8}{b}^ {2}+160715735714773856157696\,{a}^{6}{b}^{4}-160715735714773856157696 \,{a}^{4}{b}^{6}+4512413307974543474688\,{a}^{2}{b}^{8}+ 2801419437596265676800\,{b}^{10}+157002242738903580672\,{a}^{8}+ 99495970498069735145472\,{a}^{6}{b}^{2}-282658247208303920676864\,{a}^ {4}{b}^{4}+99495970498069735145472\,{a}^{2}{b}^{6}+ 157002242738903580672\,{b}^{8}+15810272187369010495488\,{a}^{6}- 123591451896180620918784\,{a}^{4}{b}^{2}+123591451896180620918784\,{a} ^{2}{b}^{4}-15810272187369010495488\,{b}^{6}-3275378007362758508544\,{ a}^{4}+10917926691209195028480\,{a}^{2}{b}^{2}-3275378007362758508544 \,{b}^{4}=0 $
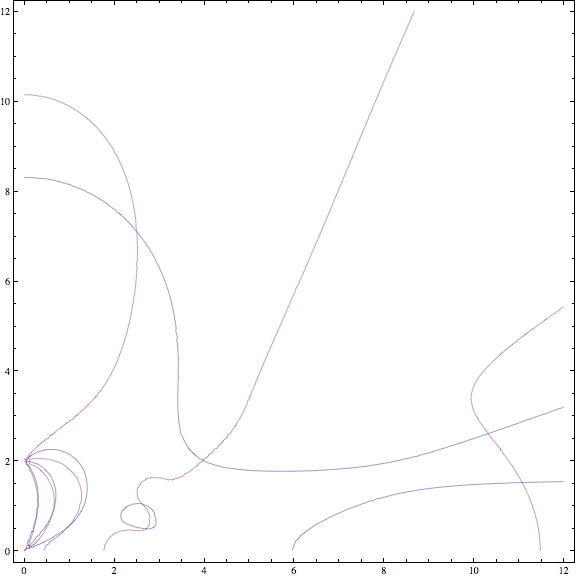
The plots of a vs b for both these equations on the same graph is:

On the graph we can see that one intersection point is near (4,2). Thus, I tried using fsolve in MAPLE to get the exact point to which MAPLE returns {a = -3.984746496, b = 2.012958923}. But, apparently, when I put back this point into the two equations I get values nowhere close to zero. I also tried using fsolve in MATLAB but no success. Somebody please help me out in solving the two equtions simulataneously.