Second derivative in coordinate invariant form

Question

To solve stationary, incompressible, inviscid and irrotational flow around a circular cylinder, I am using general coordinates. Since the flow is symmetrical, we only consider the upper half of the plane.

I derived a PDE and proper boundary conditions in the physical domain (left image) given by $G := \{(x_1,x_2 : R_1^2 \leq x_1^2 + x_2^2 \leq R_2^2, x_2 \geq 0\}$. This PDE is $\Delta \phi = 0$, where $\phi$ is the perturbation potential.

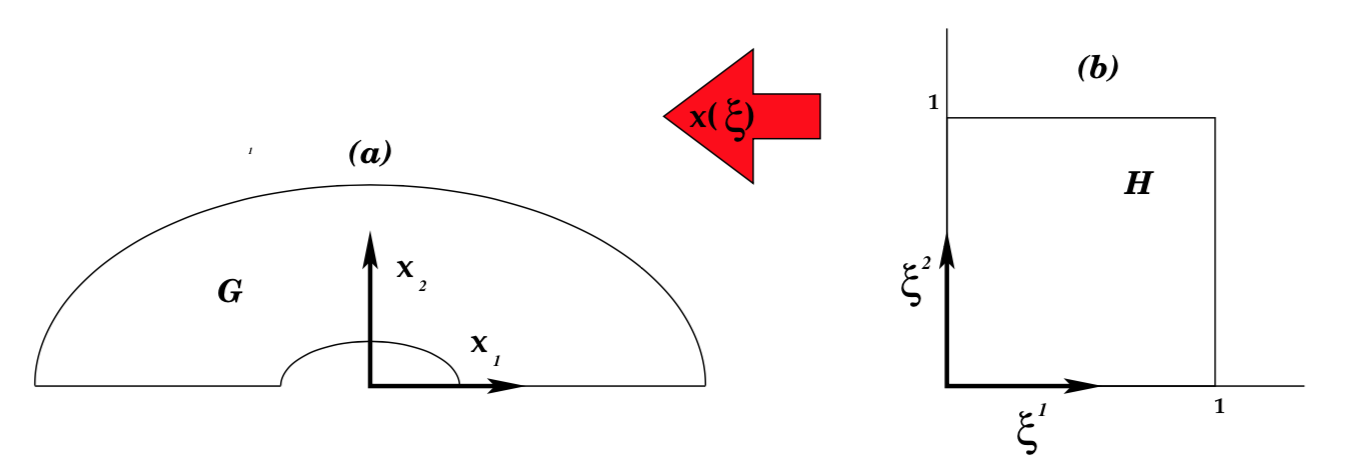
In general coordinates, the expression for $\Delta \varphi$ can be expressed as (using the Einstein summation convention) \begin{align*} \Delta \phi = \frac{1}{\sqrt{g}}\frac{\partial}{\partial \xi^\alpha}\left(\sqrt{g}g^{\alpha\beta}\frac{\partial\varphi}{\partial\xi^\beta}\right), \end{align*} where $\xi(\mathbf{x})$ is the (inverse) coordinate mapping and $g$ is the (contravariant) metric tensor.

On the cylinder itself, the BC is given by $$ \nabla\phi\cdot\mathbf{n} = -U_\infty n_1, $$ where $U_\infty$ is is the free stream velocity in the $x_1$ direction and $\mathbf{n}$ is the normal on the surface of the cylinder. In general coordinates, this BC becomes $$ \frac{\partial \varphi}{\partial \xi^2} = -U_\infty (\mathbf{a}^{(2)})_1, $$ where $\mathbf{a^{(\alpha)}}$ is the contravariant basis vector.

The other boundary condition (horizontal left and right of the cylinder) are given by (see derivation below) $$ \frac{\partial^2 \varphi}{\partial x_2^2} = 0 \ \text{ and } \ \frac{\partial^2 \varphi}{\partial x_1\partial x_2} = 0 $$ and I would like to also write this is in coordinate invariant form (in terms of the covariant/contravariant basis vectors and the metric tensor), but I have no idea where to start. Any suggestions would be helpful.

EDIT The derivation of the symmetric boundary condition is as follows. It is given that the velocity field $\mathbf{u}$ can be expressed as $u_i = \partial_i{\Phi}$, where $\Phi$ is the velocity potential function given by $$ \Phi = U_\infty x_1 + \phi. $$

I thought that on the horizontal boundary

$$ \frac{\partial\mathbf{u}}{\partial x_2} = \mathbf{0} $$

Leading to the two boundary conditions given above.

Answer 1

IMPORTANT

To keep things clear... the Laplacian operator $\triangle$ must come with ONLY one BC in each point of the boundary, this is, you can not specify 2 BC as you propose on the boundary. To se this.. I ask you sth. How many BC you need in the boundary to solve this unidimensional BVP? $$\frac{d^2 u}{d x^2}=0,\quad x\in[x_1,x_2]$$

I think the BC you are looking for this boundary is the following: $$ \vec{n}^T\sqrt{g^{\alpha\beta}}\left[\vec{grad}(\phi)\right]=0$$ Where $g^{\alpha\beta}$ is the contravariant metric tensor, $\vec{n}$ is the exterior normal vector to upper and lower boundaries and the gradient operator is expressed in the new coordinates.

EDIT Since in a symmetry plane no flow crosses this boundary we have that the BC is:

$$\vec{n}^T\vec{u}=0$$ Therefore, since $\vec{u}=\vec{grad}\,\phi$ you have the required zero normal gradient BC $$\vec{n}^T\vec{grad}\,\phi=0$$

Answer 2

The symmetry condition enforces a Neumann Boundary condition on the velocity field and reads $$n\cdot\nabla u=0$$. These are actually two equations. In any coordinate system this is done by the contraction of the covariant derivative of the velocity field with the normal vector of the boundary: $$n^i \nabla_i u^k=n^i \left(\frac{\partial u^k}{\partial \xi^i}+\Gamma^k_{il} u^l\right)=0$$. In cartesian coordinates where the coordinate lines align with the boundary, they are exactly the stated equations.

Since the velocity field $u^\alpha=g^{\alpha\beta}\frac{\partial\Phi}{\partial \xi^\beta}$ you have $$n^\gamma \nabla_\gamma g^{\alpha\beta}\frac{\partial\Phi}{\partial \xi^\beta}=n^\gamma g^{\alpha\beta}\left(\frac{\partial^2 \Phi}{\partial \xi^\beta\partial\xi^\gamma}-\Gamma^l_{\gamma \beta} \frac{\partial\Phi}{\partial \xi^l}\right)=0$$. These are two equations one for each part of the curved coordinate system with respect to the normal condition.

Further, since $\Phi=U_\infty x_1(\xi_1,\xi_2)+\phi$ you get for the derivatives of $\Phi$:$$\frac{\partial\Phi}{\partial\xi^\alpha}=U_\infty \frac{\partial x_1}{\partial \xi^\alpha}+\frac{\partial \phi}{\partial \xi^\alpha}$$.

So finally for $\phi$ this reads: $$n^\gamma g^{\alpha\beta}\left(\frac{\partial }{\partial \xi^\gamma}\left(U_\infty \frac{\partial x_1}{\partial \xi^\beta}+\frac{\partial \phi}{\partial \xi^\beta}\right)-\Gamma^l_{\gamma \beta} \left(U_\infty \frac{\partial x_1}{\partial \xi^l}+\frac{\partial \phi}{\partial \xi^l}\right)\right)=0$$.

Check this with cartesian coordinates, $x_1$ is independent of $x_2$ so these derivatives vanish. Christoffel symbols are all 0. Metric is diagonal 1, $n^1=0$, $n^2=1$: $$n^\gamma g^{\alpha\beta}\left(\frac{\partial }{\partial \xi^\gamma}\left(\frac{\partial \phi}{\partial \xi^\beta}\right)\right)=0$$ so for each component:$$ n^2 g^{1\beta}\left(\frac{\partial }{\partial x^2}\left(\frac{\partial \phi}{\partial x^\beta}\right)\right)=\left(\frac{\partial }{\partial x^2}\left(\frac{\partial \phi}{\partial x^1}\right)\right)=0\\ n^2 g^{2\beta}\left(\frac{\partial }{\partial x^2}\left(\frac{\partial \phi}{\partial x^\beta}\right)\right)=\left(\frac{\partial }{\partial x^2}\left(\frac{\partial \phi}{\partial x^2}\right)\right)=0$$.